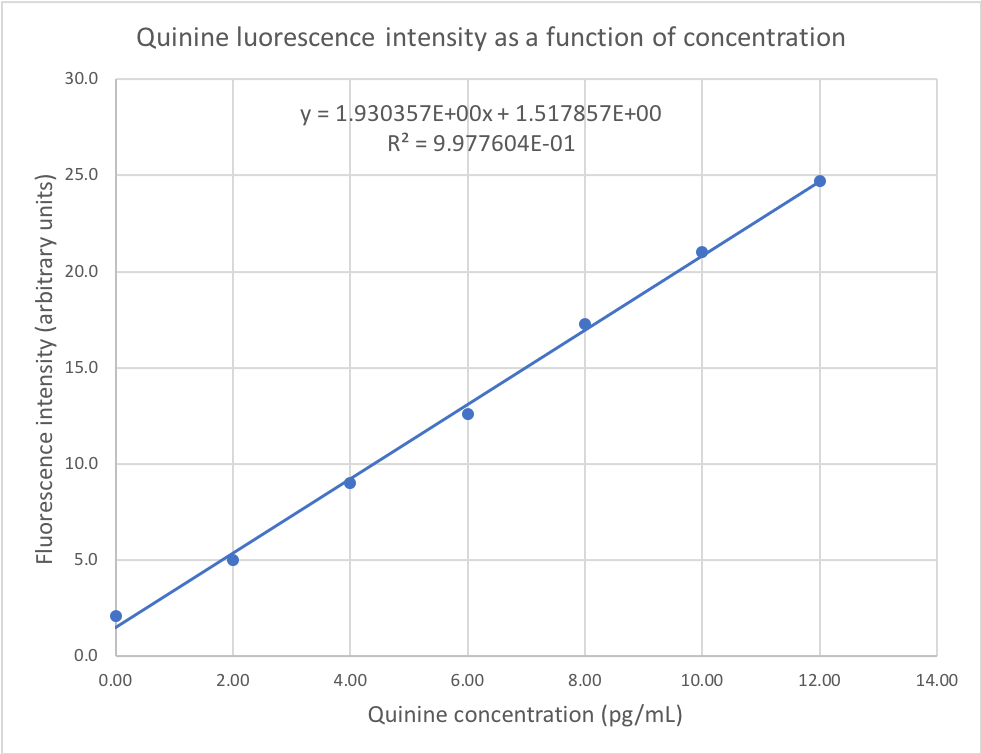
Calibration and Trendlines:
Most analytical chemistry measurements involve calibration functions that can be described using a straight line. Excel has a feature that allows you to easily display a linear trendline on your graphs, which is the best-fit straight line through your data. This feature also allows you to view the equation of the best-fit line, as well as the correlation coefficient; both of these are determined using the mathematical technique of linear regression analysis. The trendline feature provides a quick test of the linearity of your calibration data. A more complete treatment of linear regression will be provided in a later section.
A calibration curve is an equation that permits us to calculate a desired experimental result in terms of another. In the simplest form, this is given as the equation of a straight line where the x-value is the input (usually concentration) and the y-value is the output (usually the measured instrument response). As we saw in the preceding example, the relationship between response and concentration is not always linear, although it is usually possible to linearise the equation – that is, transform the data into a form that is linear, such as E versus log C.